Higher dimensional geometries. What are they good for?
Geometry originated from the study of two- and three-dimensional phenomena in the space of our perception. It took a long time until mathematicians considered spaces of four- and more dimensions. The existence of four and higher dimensional spaces was not creative commons in the beginning and even mathematicians doubted in the existence of such spaces.
When mathematicians started to look for a well-grounded basis of geometry, the mere need of higher dimensional spaces became clear, at least since Felix Klein’s notion of geometry as the theory of invariants of a transformation group. Models for various geometries or groups that are intrinsically of more than three dimensions justified the use of higher dimensional spaces, and finally, they turned out to be very useful and efficient tools in many not only inner mathematical applications.
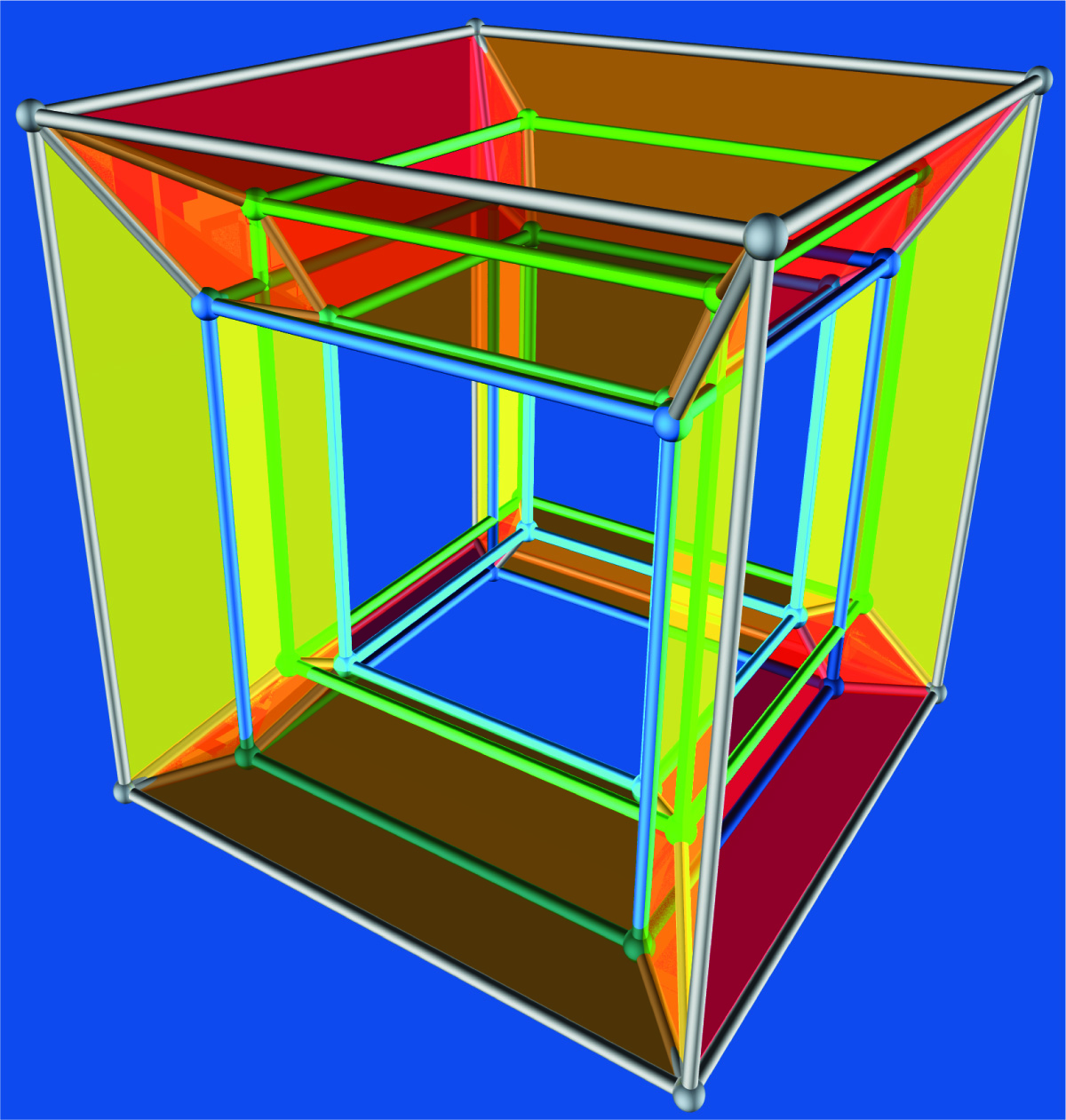
Nowadays, even undergraduate courses in mathematics and lectures for engineers treat higher dimensional spaces as somehow natural things. In this talk, we shall browse through some selected areas of geometry and its applications were higher dimensional spaces occur frequently and naturally. We shall see a variety of applications that take place in spaces of any dimension.
Generalizing concepts from lower dimensions to higher dimensions sometimes causes problems. The variety of spaces is not restricted to Euclidean or affine spaces. Non-Euclidean geometries in arbitrary dimensions occur in a very natural way. Higher dimensional models of geometries are likely to show the relations between different geometries.
Although we focus on applications that somehow justify the notion of ann-dimensional space, we shall also see some nice examples that are purely mathematical in nature. Abstract structures should not only be seen as the toys of mathematicians playing in their ivory towers. These structures are of a certain beauty. The choosen examples shall illustrate the usefulness and effectiveness of so called higher (dimensional) geometries.